In the analysis of rotating devices or solids, superior predictions can be obtained when the flow field is computed within the relative reference frame (a reference frame that rotates with the rotating device). To accommodate multiple rotating devices, which may have a variety of rotating speeds and/or axes, it is best to create a rotating region that surrounds each rotating device. These rotating regions are then surrounded by a non-rotating region.
In the following sketch, each rotating device (in red) is surrounded by a circular fluid region (in green). The outer region enclosed in the box is a stationary region.
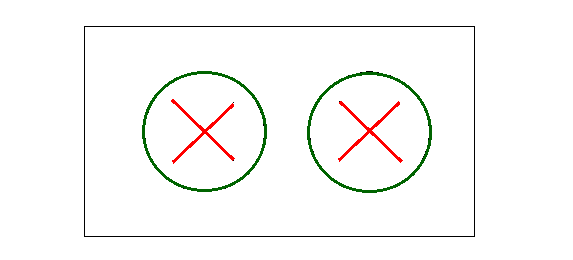
These fluid regions rotate with the same angular speed as the rotating device that they surround. Within the rotating regions, all computations are performed in the relative frame of reference. Within the stationary regions, all computations are performed in the inertial frame of reference.
When fluid motion is computed in a relative frame, some addition accelerations must be considered:

at = tangential acceleration

acentrip = centripital acceleration
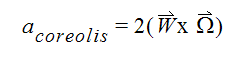
acoreolis = coreolis acceleration
where, the velocity in the inertial frame is related to the velocities in the relative frame using,

These additional momentum acceleration source terms are only required in the rotating fluid zones.
At each time level, the rotating components and the fluid meshes that surround them, will rotate by a few degrees. At a given rotational speed, the angle of rotation is computed as,

where the rotating speed is given in revolutions per minute (RPM). With the rotating mesh in it’s new position, all scaler quantities are mapped from the rotating side to the non-rotating side of the sliding mesh interface using geometric interpolation between the two mesh systems. Information is transferred on an “advection” basis. In other words, when examining each fluxing face on the rotor-stator interface, flow going from the rotor to the stator, will advect quantities in the direction of flow. Likewise, flow from stator to the rotor will advect information from the stationary mesh to the rotating mesh. In the case of connecting the momentum transferred through the sliding mesh zone, the process is very similar, except a transformation is required to either add or subtract the “omega cross r” quantity when transforming from the relative to absolute frame or visa-versa.
When the speed of a rotating device is not known, conservation of angular momentum is used to predict the angular speed over time.

In this equation, hydraulic torque (arising from pressure and shear forces acting on the surfaces of the rotating device) and externally applied torques will either accelerate or decelerate the rotating device. The inertia of the rotating device is given the symbol, I. Note that for a free spinning device, which does not have an applied external torque, that the device will accelerate until the induced hydraulic torque vanishes.