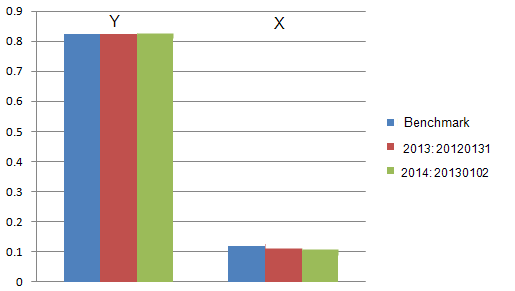Laminar Thermal-Driven Cavity

Analysis Characteristics

• Steady-state
• 2D internal flow
• Laminar
• Incompressible
• Natural convection

Reference

Davis, G. De Vahl and Jones, I.P., “Natural convection in a square cavity: a comparison exercise”, Inter. Jour. for Num. Meth. in Fluids, 3, (1983).

Problem Description

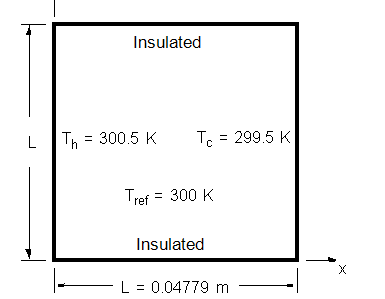
Temperature and velocity distributions are calculated for laminar, buoyancy-driven flow in a square cavity. The top and bottom walls are insulated, and the left and right walls are at fixed temperatures differing by 1 K.

The Rayleigh number is computed from:

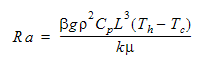
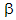• is the coefficient of volumetric expansion, defined as: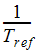

• g is the acceleration of gravity
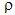• is the density
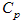• is the specific heat
• L is the length of the cavity
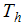• and 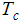are the temperatures of the left and right walls, respectively
• k is the conductivity of the fluid
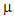• is the viscosity.
• Here, the Rayleigh number is 10,000.

This problem is analyzed to verify the fluid flow and heat transfer modeling capabilities of Autodesk Simulation CFD. Accuracy is assessed by comparing velocity components at specific locations in the cavity. Velocities and coordinates are normalized in accordance with Davies, et al. (1983) as follows:

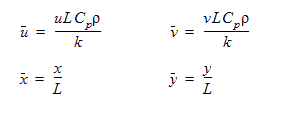
Geometry and Boundary Conditions

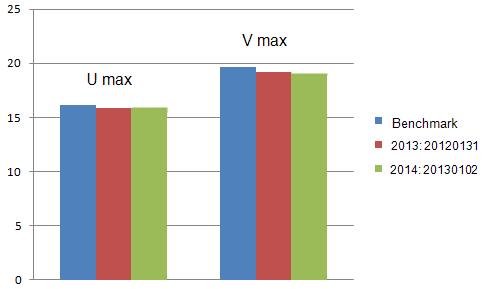
Results

Using the expressions defined above for 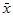, , 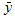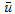and 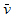, the following results are computed:

	Benchmark	2013: Build 20120131	% Error	2014: Build 20130102	% Error
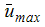
	16.178	15.905	1.686	15.862	1.952
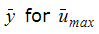
	0.823	0.826	0.394	0.826	0.404
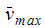
	19.617	19.488	0.653	19.458	0.809
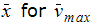
	0.119	0.114	4.188	0.113	4.373