The Puck criterion (Ref. 6 and 7) identifies fiber failure and inter-fiber failure in a unidirectional composite. It further separates inter-fiber failure into three different physical modes and further separates fiber failure into two different physical modes. The general form of the Puck criterion utilizes the full 3-D state of stress and strain, but Helius Composite's implementation considers only in-plane stresses and strains consistent with Classical Laminate Theory.
- Composite Material Type - Either a carbon fiber reinforced polymer (CFRP) or a glass fiber reinforced polymer (GFRP) must be specified.
- Fiber Material Type - For lamina materials not defined using the micromechanics module (Calculating New Lamina Properties from Micromechanics), you must identify the fiber material used in the composite lamina.
Fiber Failure
The Puck criterion recognizes two different modes of fiber failure, the first being a tensile failure, and the second being a compressive "fiber kinking" failure. The tensile fiber failure criterion is
and the compressive "fiber kinking" failure is
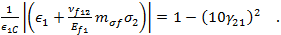
In the above fiber failure criteria,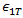 
and 
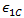are the composite strains corresponding to composite longitudinal tensile and compressive failure, respectively, 
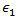is the uniaxial strain in the composite, 
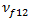is the longitudinal Poisson ratio of the fiber, 
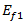is the longitudinal tensile modulus of the fiber, 
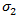is the transverse stress of the composite, 
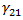is the longitudinal shear strain in the composite, and 
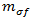is intended to capture the differences in the transverse stresses in the fiber and matrix. For carbon fibers 
, and for glass fibers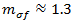 
. In the above equations, the tensile equation is evaluated if 
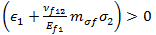, and the compressive criterion is evaluated if 
.
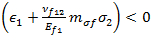Inter-Fiber Failure (Matrix Cracking)
In the Puck criterion, inter-fiber failure encompasses any matrix cracking or fiber/matrix debonding. The Puck criterion recognizes three different inter-fiber failure modes, referred to as modes A, B, and C. These inter-fiber failure modes are distinguished by the orientation of the fracture planes relative to the reinforcing fibers.
- Inter-Fiber Failure Mode A
- Mode A corresponds to a fracture angle of 0°. The criterion is invoked if the transverse stress in the composite is greater than 0 (thus indicating a transverse crack perpendicular to the transverse loading).
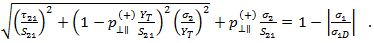
- Inter-Fiber Failure Mode B
- Mode B corresponds to a transverse compressive stress (inhibiting crack formation) with a longitudinal shear stress which is below a fracture resistance (coupled with empirical constants).
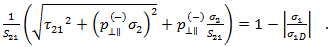
- The above criterion is evaluated if
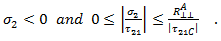
- Inter-Fiber Failure Mode C
- Mode C corresponds to a transverse compressive stress (inhibiting crack formation) with a longitudinal shear stress which is significantly large enough to cause fracture on an inclined plane to fiber axis. Within the context of Classical Laminate Theory, we do not need to define the fracture angle, as it is irrelevant for failure predictions involving only in-plane stresses and strains (and no degradation of material properties). The failure criterion for Mode C is
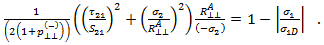
- The above criterion is evaluated if
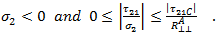
Description of Coefficients and Terms used in the Inter-Fiber Failure Criteria
We will make use of commonly accepted notation, e.g.,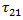 
is the composite longitudinal shear stress, 
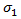is the composite longitudinal normal stress, 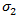is the composite transverse normal stress, 
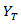is the composite transverse tensile strength, and 
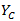is the transverse compressive strength. 
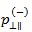and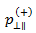 
are the slopes of the 
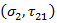fracture envelope. To establish the connection between 
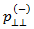and 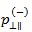, Puck assumes the following relationship holds
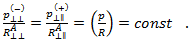
Therefore, 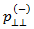is given by
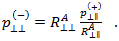
Within the context of the in-plane stresses and strains of the Classical Laminate Theory,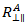 
can be defined as 
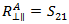which allows Puck to express 
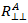as
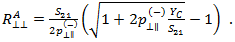
Now we must define the values for 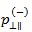and 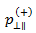. Puck and Mannigal (2007) provide the following recommended values for 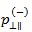and .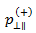
Puck also defines 
as
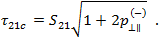
Finally, we must define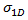 
. That is a "degraded" stress in the composite allowing for pre-fiber failure breakage of individual fibers, which causes localized damage in these areas in the form of microcracking and debonding. To account for this weakening effect, Puck degrades the fracture resistances (R) by a weakening factor 
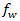. Puck defines two equations for this. The first is for the generalized weakening factor.
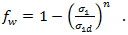
The second is to give another expression of the weakening factor in order to keep the fracture conditions homogeneous and of first degree with respect to the stresses.
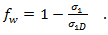
For the in-plane stress states considered by Helius Composite, these two should be equal since there are no iterative calculations on fracture planes being performed. Therefore,
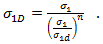
Based on the recommendations of Puck, Helius Composite uses n=6 for the exponent and empirically compute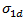s 
as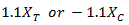 
depending on the sign of .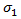