Derive basic equations for closed linear dimension chains from the following image.

a = 32 ± 1.5 mm, b = 8 ± 1 mm
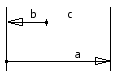
Increasing/decreasing elements
If the increasing element increases, the closing element also increases. If the decreasing element increases, the closing element decreases, if other elements of the chain remain constant.
|
Closing element: |
1. c = a - b |
|
Upper limit dimension of the closing element: |
2. c max = a max - b min |
|
Lower limit dimension of the closing element: |
3. c min = a min - b max |
|
Tolerance of the closing element (subtracting equation 3 from equation 2) |
4. c max - c min = a max - a min - (b min - b max ) |
|
Such as |
5. T c = T a + T b |
In general, linear chain tolerances satisfy the following equation.
![]()
Similarly, equations 2 and 3 can be written in a general form.
![]()
![]()
Upper limit dimension of the closing element equals a difference of the sum of upper limit dimensions of increasing elements and the sum of lower limit dimensions of decreasing elements.
Lower limit dimension of the closing element equals a difference of the sum of lower limit dimensions of increasing elements and the sum of upper limit dimensions of decreasing elements.
Nominal dimension of the closing element can be expressed using equation 1, as in the following equation.
![]()
Upper limit deviation of the closing element.
![]()
Lower limit deviation of the closing element.
![]()