Calculations are performed according to the Coulomb failure wedge theory.
Soil pressures
The following 3 characteristic (limit) modes of soil actions (pressures) on a retaining structure can be distinguished:
- Static pressure Po - If a retaining element has not been displaced
- Active pressure Pa - If a retaining element has been displaced, because of the action of external forces, in the direction compatible with the pressure caused by soil
- Passive pressure Pp - If a retaining element has been displaced because of the action of external forces, in the direction opposite to the pressure caused by soil
The pressure is directly related to the displacement of a retaining structure. The displacement is determined using the rotation of element ρ, which is assumed to approximately equal the ratio f/H (wall top displacement / element height).
Pa <- ρ ≤ ρa
Po <- ρ = 0
Pp <- ρ ≥ ρp
Where ρa and ρp are the limit pressures for the corresponding modes
It is assumed that there is a linear relation between the following pressures:
Ka * P < Ko * P < Kp * P,
Where P is a certain pressure, which in the simplest case, is equivalent to hydrostatic pressure. Intermediate pressures are contained between the limit pressures.
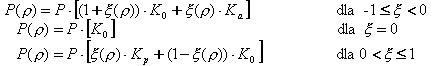
Where ξ from the interval <-1,1> corresponds to the linearly standardized variable from the interval <ρa, ρp>.
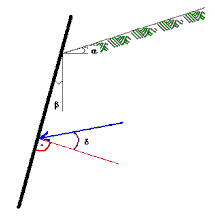
Soil pressures depend on the soil unit weight γ, natural slope angle ϕ, inclination of a retaining element β, and the backfill inclination α. The effect of the wall-soil friction angle δ is disregarded.
- Hydrostatic pressure equals the weight of soil above the plane being analyzed. For layered soils (n layers above a given point), it amounts to:
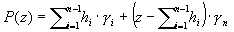
- Pressure influenced by the presence of other objects. This type of pressure occurs, if in the vicinity of a retaining element, there are other objects, which reduce the value of soil pressure. This type of object prevents the P(z) pressure to be fully identified with the hydrostatic pressure. It is equivalent to the hydrostatic pressure until a certain zgr depth is reached, afterwards the P(z) pressure is constant:

- Static pressure is pressure caused by the soil when the displacements of the retaining element reach zero. This pressure equals the hydrostatic pressure multiplied by the coefficient Ko: Po(z) = Ko * P(z), where Ko = 1 - sinα.
- Active pressure is pressure caused by soil, when a retaining element has been displaced because of the action of external forces by a value which is at least equal to the limit value ra in the direction compatible to the pressure caused by soil. This pressure equals the hydrostatic pressure multiplied by the coefficient Ka: Pa(z) = Ka * P(z), where

- Passive pressure is pressure caused by soil, when a retaining element has been displaced because of the action of external forces by a value which is at least equal to the limit value ρp in the direction opposite to the pressure caused by soil. This pressure equals the hydrostatic pressure multiplied by the coefficient Kp: Pp(z) = Kp * P(z), where
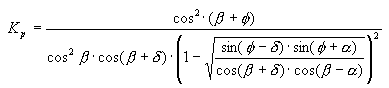
- Intermediate pressures occur if the displacement of the retaining element is contained in the interval ρa < ρ < ρp and ρ≠0. It is a combination of static pressure and active or passive pressure:
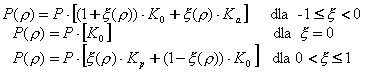
Pressures induced by loads applied to soil surface
It is assumed, that the pressure resulting from loads applied to soil is obtained by multiplying P'(z) pressure by the appropriate K coefficient (Ko, Ka, Kp or their combination), analogously as for soil pressure.
Depending on the code selected, the following methods and factors of load distribution are used:
Uniform
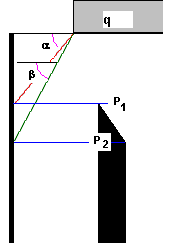
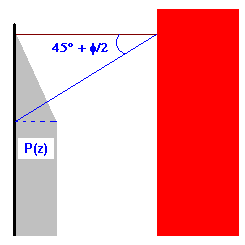
PN-83/B-03010: α=Φ, β=45+Φ/2 as well as P1= 0 and P2 =K q
PN-85/S-10030: α=Φ, β=45+Φ/2 as well as P1=K q and P2 =K q
SETRA (France): α=Φ, β=45+Φ/2 as well as P1= K q and P2 =K q
RD 31.31.27-81 (Russia): No algorithm
Linear
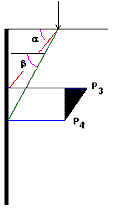
PN-83/B-03010: α=Φ, β=45+Φ/2 as well as P3= K Q and P4 =0
PN-85/S-10030: α=Φ, β=45+Φ/2 as well as P3= K Q and P4 =K Q
SETRA (France): α=Φ, β=45+Φ/2 as well as P3= K Q and P4 =K Q
RD 31.31.27-81 (Russia): α=45+Φ/2, β=45+Φ/2 as well as P3= K Q and P4 =K Q
Evenly distributed
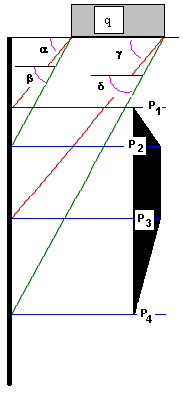
PN-83/B-03010: α=γ=Φ, β=δ=45+Φ/2 as well as P1=P4=0 and P2=P3=K q
PN-85/S-10030: α=Φ, β=45+Φ/2 as well as P1=P4= P2=P3=K q
SETRA (France): α=Φ, β=45+Φ/2 as well as P1=P4= P2=P3=K q
RD 31.31.27-81 (Russia): α=45+Φ/2, β=45+Φ/2 as well as P1=P4= P2=P3=K q
Soil cohesion
Forces of soil cohesion are ignored when calculating soil pressure. The cohesion is not always taken into account in calculations, because it has a favorable effect on structures. It is a force which causes an excavation to be preserved even without a retaining element. The cohesion acts in the direction opposite to the displacement of a retaining element, thus reducing the action of the soil on a wall. A value of pressure due to cohesion is constant for a given soil and equals:
Where c denotes soil cohesion and coefficient Kc equals:
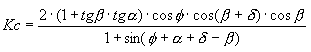
Cohesion is considered only for cohesive soils.