Input Parameters for Defining Cohesive Constitutive Relations

Review the constants used to define the cohesive material constitutive relations.

The cohesive constitutive relations are formulated as traction/separation laws that define the transverse normal traction and transverse shear traction of the cohesive material in terms of the relative displacements between the upper and lower surfaces of the cohesive element. These traction/separation laws for the cohesive material are defined via a small number of user-specified constants described below.

Stiffness (Knn, Kss, Ktt)

The stiffness of the cohesive material is defined via three constants (Knn, Kss, Ktt) which are input as material moduli of the cohesive material (units of force/area). Knn is the original un-damaged transverse normal modulus of the cohesive material, while Kss and Ktt are the original un-damaged transverse shear moduli of the cohesive material. Within the cohesive UMAT, these material moduli (Knn, Kss, Ktt) are scaled via the thickness of the cohesive element to express stiffness in units appropriate for relating traction to relative displacements of the upper and lower surfaces of the cohesive element.

Initiation Strengths (Sn, Ss, St)

Prediction of damage initiation in the cohesive material is traction-based and depends entirely on the transverse normal traction (t n ) and the transverse shear tractions (t s and t t ) borne by the cohesive material. For a pure transverse normal loading of the cohesive material, Sn is the normal traction (t n ) at which damage initiation occurs. For pure transverse shear loading of the cohesive material, Ss and St are the shear tractions (t s and t t ) at which damage initiation occurs.

Helius PFA provides two different traction-based criteria to signal delamination initiation (or damage initiation) of the cohesive material, namely, the Max Stress Criterion and the Quadratic Stress Criterion (described later).

Dissipation Energy of Delamination

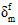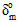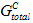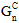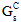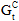( deltam sup0 - ), ( gtotal sup0 ), or ( gn sup0 , gs sup0 , gt sup0 , α)

There are three different methods for specifying the amount of energy dissipated by the cohesive material during the delamination process. These methods are referred to as the total displacement model (where you specify 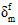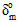deltam sup0 - ), the total energy model (where you specify 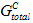gtotal sup0 ), and the mixed mode power law (where you specify 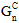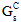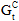gn sup0 , gs sup0 , gt sup0 , α).