Woven Composites
Examine woven composite materials using KTF.
In woven lamina, microcracking of the matrix pocket during fatigue loading has a very insignificant effect on the load bearing response of the lamina [22-24]. Therefore, we neglect any failure from microcracking in the matrix pocket as it does not play a major role in the fatigue process of woven laminae.
It is proposed that meta-delaminations have a pronounced effect on material hysteresis through frictional effects of tows wearing on one another during a fatigue loading [25-26]. It is also proposed that these delaminations have the capability to decouple the tows, allowing stress redistribution during the fatigue loading. For the sake of simplicity, we neglect the effects of meta-delaminations on the fatigue solution for now. We also assume that final failure of the woven microstructure initiates through matrix cracking perpendicular to the fibers. Once the matrix failure initiates within the tow bundle, the final failure of the tow is closely followed.
The assumptions listed above used to idealize the fatigue failure process can now be proposed as a sequence of the following events:
- Transverse cracking in matrix constituent within bundles from fatigue loading
- Longitudinal cracking of the matrix constituent from fatigue loading, resulting in fiber failure
Finally, experimental data rarely reports the initiation of transverse cracking in bundles due to fatigue loadings. The only information reported is the cycles to failure at the applied stress level. Therefore, we assume only longitudinal cracks contribute the final fatigue failure of plain woven materials. While this may seem a gross simplification, woven composite materials are designed for transverse cracks within the bundles. The architecture of the tows provides a "fiber" response in any in-plane direction of the lamina.
On-Axis
Any loading in the plane of a plain woven lamina eventually results in a catastrophic failure event through fiber breakage. We have previously stated that ultimate failures in fatigue loading due to longitudinal cracks stem from cracks within the matrix constituent which bridge fibers. Shortly after the appearance of the longitudinal cracks, the fibers fail due to the large amount of stress concentrations present within the fiber constituent.
In theory, the on-axis effective stress developed for unidirectional materials could be applied to the woven microstructure as well. However, the use of volume averaged stresses presented on the previous page "filters out" self-equilibrating stresses. In a plain woven composite this means that a pure shear loading would result in only the shear component of stress present in the matrix constituent, as the other stress components are self-equilibrating. A pure shear loading would not cause any effective stress for a final fatigue failure, even though pure shear loadings have been shown to cause fatigue failures of woven lamina [22]. Therefore, we modify Eq. 44 for plain woven composites as:

where

Eq. 47 can be modified to produce a failure index instead of an effective stress as

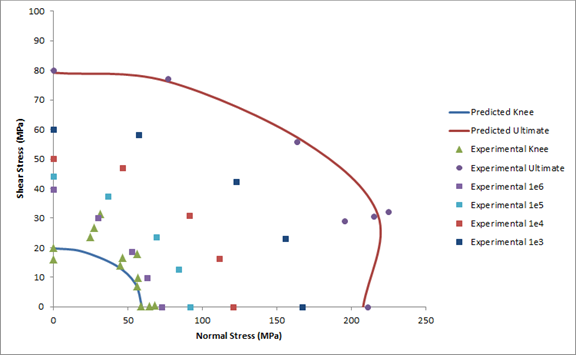
The values of A i are determined from three composite static failure tests: longitudinal tension, longitudinal compression, and in-plane shear. The failure criteria presented in Eq. 47 has been verified against experimental data and the results are presented below, labeled as Predicted Ultimate.