1. Standard Calculation Procedure
Checks joint strength by direct comparison of calculated normal, shear, or resulting reduced stress with the allowable stress by using a standard calculation procedure. With a view to the type of the weld joint, design and loading (that is, with respect to acting stress), strength check can be defined with the following formulas.
σ ≤ σ Al , τ≤τ Al , σ R ≤σ Al
where the formulas for allowable loading of the weld joint are (with respect to the required safety):
s Al = S Y / n s or t Al = S Y / n s .
The size of allowable stress, and after the required minimum joint safety, depends on the type of acting stress. For example, the type, design, and loading of the weld joint.
This method is for experienced users who can estimate correctly (according to type, design, and weld loading) the required minimum size of safety factor of the weld joint.
2. Method of Comparative Stresses
Allowable stress is compared with auxiliary comparative stress, which is determined from the calculated partial stresses by using conversion factors of the weld joint when the strength check is carried out with this method. Strength check can be described by the s S ≤ s Al formula, in which allowable loading of the weld joint is s Al = S Y / n s .
While using empirical conversion factors, effects of different stress types to weld joint safety are included in the calculated comparative stress. You can work with only one value of the safety factor, regardless of the type, design, and loading of the selected weld joint. The recommended minimum value of the safety factor for the method of comparative stresses is within the n S =< 1.25...2> interval.
This method is for less experienced users.
Weld Joint Calculation Parameters
1. Total versus throat (active) weld length
The size of throat area of the weld has a substantial effect on strength of the weld joint. Generally this value is a multiple of the weld length and height (thickness). For eventual reduction of the area at the beginning and at the end of the weld, in more precise calculations it is better to use only the weld part for the throat length that has the given area.
The weld throat length is determined by using L' = L - 2s formula for butt welds or L' = L - 2a for fillet welds,
where:
|
s |
less thickness of welded parts. |
|
|
a |
fillet weld height. |
Recommended size of the throat (active) length of fillet weld is in the L' =< 3a...35a> range.
This switch has no effect for peripheral welds, where the throat weld length is always the full weld length.
2. Thickness of flange and web is ignored
Thickness of flange and web can be ignored in calculations of beams with T or I section, connected with fillet welds. For standard sections, the ratio of flange or web thickness and beam width is small and for this reason the calculation is sufficiently precise if thickness is ignored.
We recommend that you switch off this calculation option for precise calculations or for special sections (with a greater flange or web thickness).
3. Distribution of shear stress is considered
For beams joined by fillet weld and loaded with shear force and for more precise calculation, we recommend that you use the theory of shear stress distribution in the loaded section and to consider only welds that carry the shear force within the calculation. According to this theory, the shear force is carried only by welds parallel with stress direction. Shear stress is then calculated by using the formula t = F Y / A s , where:
|
F y |
shear force. |
|
|
A s |
reduced throat of weld group. |
4. Only positive stress value from bending moment is considered
For beams joined by filled welds and loaded with bend moment, normal stress is originated in the weld. The following is an image of the stress diagram.
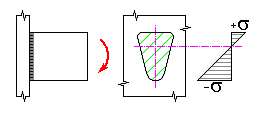
The maximum stress is originated in the outer points of the weld group, the most distant places from the neutral axis. For welds, symmetrical along the neutral axis the size of these stresses is identical. For nonsymmetrical welds, pressure stress might be greater. Normally the program tests a greater value from these peaks during strength check, regardless of the stress direction, which is pressure stress in this case.
When loading capacity of the weld joint is considered, tensile stress has substantially greater significance for such welded beam. This switch suppresses the pressure stress check and allows a check of the maximum tensile stress value only, even if the pressure stress is greater in the weld.
This switch is applicable only for static calculation because there is no difference between positive or negative value for fatigue calculation and the calculation is always controlled by maximum stresses in the weld.