This program produces a preliminary design for the diametral clearance of the specified bearing journal diameter and speed. The value of the diametral clearance is designed using a relative diametral clearance, which is calculated according to empirical formula:
where:
| Ψ |
relative diametral clearance [-] |
|
|
v H |
circumferential journal speed [m s -1 ] |
The relative diametral clearance is an important design parameter that affects the bearing properties. Its range is usually 0.0005 to 0.004. Small values of relative diametral clearance are suitable for bearings with high specific pressure, working at slow sliding speeds and vice versa.
With an increasing value of relative diametral clearance, the bearing load capacity falls and a risk of journal vibrations and cavitation of the bearing lining increase. The sliding journal speed has a most significant effect on the radial clearance selection. The selection is made according to the lining material and practice:
|
Babbitt |
(0.5 ~ 1) .10 -3 |
|
Bronzes |
(0.8 ~ 2) .10 -3 |
|
Aluminum alloys |
(1.2 ~ 2.5) .10 -3 |
|
Cast iron, graphite |
(2 ~ 3) .10 -3 |
|
Plastics |
(1.5 ~ 10) .10 -3 |
Lower values are selected for narrow and precise bearings, because edge loading does not occur.
Reduction of the inner bushing diameter due to pressing into the bearing housing:
While pressing a bushing into the bearing housing with relative interference:
some contact pressure arises:
where:
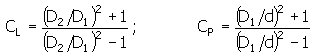 |
Recommended size of relative interference:
ϑ ≈ 1.3. 10 -3 - bearing housings from aluminum alloy;
ϑ ≈ 0.6. 10 -3 - bearing housings from cast iron or steel.
Change of diametral clearance, due to pressing the bushing, is determined in the following equation:
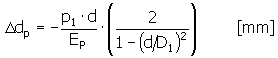
Change of the diametral clearance due to radial temperature gradients:
Bearing parts expand due to the heat build up during operation. Under the influence of radial temperature gradients, a change of radial diametral clearance happens and its value is:
Δ φ T = (α L - α H ) (1 - B) (T V - T U ) - 0.6 (α L ΔT rL - 0.75 α H Δ T rH
where:
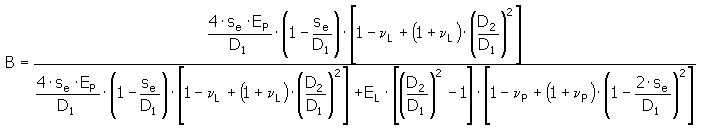 |
while the bushing effective thickness is: s e = (D1 - d s V ) / 2 [mm]
the radial temperature gradient between the outer bearing surface and sliding surface is:
ΔT rL ≈ 5 ... 15 [°C]
the radial temperature gradient between the sliding surface and shaft center is:
ΔT rH 11.0pt ≈ 2 ... 5 [°C]
Change of diametral clearance due to radial temperature gradients is determined in the following equation:
Δd T =Δφ T d [mm]
Meaning of used variables:
|
d |
Journal diameter [mm]. |
|
D 1 |
Inner diameter of bearing body [mm]. |
|
D 2 |
Outer diameter of bearing body [mm]. |
|
Δd p |
Change of diametral clearance from clamping the bushing evoked by pressing [mm]. |
|
ΔdT |
Change of diametral clearance from radial temperature gradient [mm]. |
|
Δd 1 |
Mean value of interference from pressing the bushing into the bearing body [μm]. |
|
E L |
Elasticity modulus of bearing body material [MPa]. |
|
E p |
Elasticity modulus of bushing material [MPa]. |
|
S e |
Bushing effective thickness [mm]. |
|
S v |
Thickness of bushing lining [mm]. |
|
T U |
Temperature of the bearing nearest surrounding [°C]. |
|
T v |
Lubricant mean temperature at the bearing outlet [°C]. |
|
ΔT |
Radial temperature drop between the outer bearing surface and the sliding surface [°C]. |
|
ΔT rH |
Radial temperature drop between the outer bearing surface and the sliding surface [°C]. |
|
ΔT rL |
Radial temperature drop between the sliding surface and the shaft center [°C]. |
|
α L |
Factor of thermal expansibility of the bearing body [°C -1 ]. |
|
α H |
Factor of thermal expansibility of the bearing journal material [°C -1 ]. |
|
ν L |
Poisson's ratio of the bearing body material [-]. |
|
ν p |
Poisson's ratio of the bushing material [-]. |
| υ |
Relative interference [-]. |