Thermal power originates in the bearing due to friction losses. The power is in balance with the heat conducted from the bearing. The heat is transferred from the bearing by passing from the bearing housing surface and shaft to the ambient environment. The passage of heat is made by conduction and emissions, and primarily it passes to the flowing lubricant.
A major part of the originated heat (more than 75 % is estimated) is conducted from the bearing by the lubricant. Naturally the lubricant is therefore warmed from an input temperature up to the temperature at the bearing outlet, while flowing through the bearing. The task of the bearing thermal balance calculation is to find a mean lubricant temperature at the bearing exit at which the selected lubricant has in balance the heat power originated by friction with the heat conducted from the bearing.
The quantity of heat, conducted from the bearing by the lubricant, depends on the lubricant density and viscosity. Because both the lubricant density and viscosity are expressively changed with just the temperature change, it is necessary to use a method of successive approximation for finding an outlet lubricant temperature. The expected outlet lubricant temperature, proposed by the user, is used as a basis for the iteration procedure of the calculation. The iteration calculation is ended when the difference between the designed temperature and the calculated temperature is less than 2 deg. C. A greater difference cannot be ignored, because it results in a substantial change of oil viscosity and load capacity for the oil layer.
All calculation equations, used for the bearing thermal balance, are presented in the following text:
Side oil outflow due to hydrodynamic pressure
If the bearing is not edge tightened, the oil leaks out through the edge lubrication gap, due to the hydrodynamic pressure. The volume of oil leakage is:
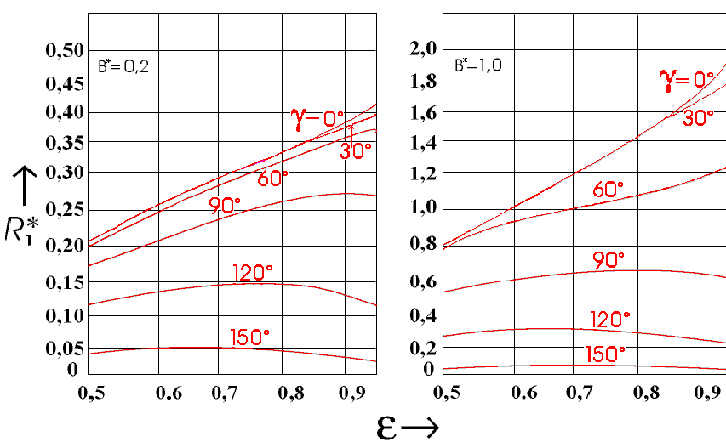
V z = 0.125 R * 1 ε d 3 φω 10 -3 [cm 3 s -1 ]
where the R * 1 outflow characteristic number is determined from the respective diagram for bearing relative width, relative journal eccentricity and the angle of lubricant inlet.
Oil outflow due to inlet pressure
If the oil is supplied to the bearing with the inlet pressure, the oil outflow is increased by the respective value. The volume of oil, escaped due to the inlet pressure, is determined at the bearings lubricated by a radial (that is, circumferential) slot, with the following equation:
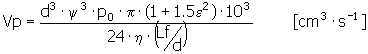
at the bearings lubricated through a lubrication hole or axial lubrication slot:
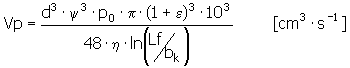
Quantity of circulated oil
Part of the oil, which was in the vacuum layer, returns back to the pressure layer and stays in circulation. Its quantity
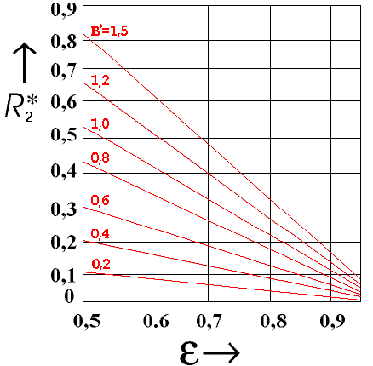
V z = 0.125 R * 2 ε d 3 φω 10 -3 [cm 3 s -1 ]
depends on the R * 2 characteristic recirculation number, which is found in the diagram according to bearing relative width and relative journal eccentricity:
Total quantity of lubricant which is supplied into the bearing
The total quantity of lubricant is determined according to the following conditions:
- Lubricant supply without pressure and with recirculation. Only the loss due to side outflow is added.
V = V z [cm 3 s -1 ]
- Lubricant supply without pressure and without recirculation. The oil passed through the lubrication layer is wiped or flushed because of the cooling.
V = V z + V u [cm 3 s -1 ]
- Pressure oil inlet with recirculation.
V = V z + V p [cm 3 s -1 ]
- Pressure oil inlet with wiping or flushing warmed-up oil.
V = V z + V p + u [cm 3 s -1 ]
Filling the vacuum part of slot
The thin oil layer, which is continuous in the pressure part of the lubrication layer, starts to disintegrate and the bearing slot simultaneously starts to fill with the air released from oil and sucked from the bearing edges and is also filled by oil vapor. The more the thin oil layer vaporizes in the vacuum part, the more the friction losses are reduced. Relieving or removing the non-loaded bushing part contributes to the disintegration of the thin oil layer. On the contrary, the complete filling of the lubrication slot happens when the following two conditions are true:
p o > 0.4 [MPa]
![]()
Friction losses are the highest in these conditions.
Friction factor
For the partial filling of lubrication slot:
μ = φΜ * 1 [-]
For the full filling of lubrication slot:
μ = φΜ * 2 [-]
Where M * 1 , M * 2 characteristic friction numbers are determined from a diagram for relative bearing width and relative journal eccentricity:

The power lost in the bearing by friction
![]()
Friction power conducted to the surroundings is
P U = 3.5 π d L α W (T V - T U ) 10 -6 [W]
where the factor of heat removal is
α W = 12 + 8 ν V / 1.2 [W m -2 K -1 ] for ν V ≤ 1.2 m s -1
![]()
for ν V ≤ 1.2 m s -1
Specific thermal capacity of the lubricant for the mean lubricant temperature at the bearing outlet is
c T = 4.588 T V - 5.024.10 -3 ρ 2 20 + 7.1156 ρ 20 - 619.646 [J kg -1 K -1 ]
Lubricant density for the mean lubricant temperature at the bearing outlet is
ρ T = ρ 20 - 0.65 (T - 20) [kg m -3 ]
Warming-up the lubricant between inlet and outlet is
![]()
where a factor of internal cooling expresses the conducted relative heat from the bearing is:
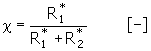
Mean that calculated lubricant temperature at the bearing outlet is
T v = T o + ΔT [°C]
Meaning of used variables:
|
b k |
lubrication hole diameter or the length of axial lubrication groove [mm]. |
|
d |
journal diameter [mm]. |
|
Δd |
diametral clearance [mm]. |
|
F |
loading force [N]. |
|
L |
bearing width [mm]. |
|
L f |
active bearing width [mm]. |
|
p 0 |
lubricant input pressure [MPa]. |
|
T U |
temperature of the bearing nearest surrounding [°C]. |
|
T V |
lubricant mean temperature at the bearing outlet [°C]. |
|
T 0 |
lubricant input temperature [°C]. |
|
v H |
circumferential speed of bearing journal [m s -1 ]. |
|
v V |
speed of air flow [m s -1 ]. |
|
α W |
factor of heat removal [W m -2 K -1 ]. |
| ε |
relative journal eccentricity [-]. |
| η |
lubricant dynamic viscosity for its mean temperature at bearing outlet [Pa s]. |
|
ρ 20 |
lubricant density for the temperature of 20 °C [Kg m -3 ]. |
| χ |
factor of internal cooling [-]. |
| φ |
relative diametral clearance [mm]. |
| ω |
hydrodynamically effective angular speed of bearing journal [s -1 ]. |