The first step in the adjustment of a horizontal survey is the calculation of C, the coordinate matrix, with the approximate coordinates for all stations.
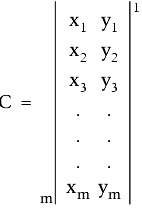
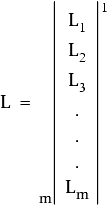
Next, the L matrix is calculated based on the calculated observations derived from the coordinate matrix, C, and the measured observations. The following formula is used to determine each Li observation value:
where:
- Omeas = the observation as measured
- Ocalc = the observation as calculated
The L matrix appears as follows:
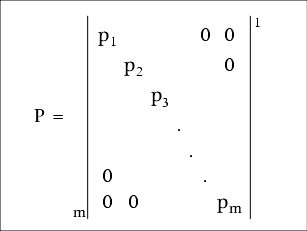
Next, the diagonal weight matrix, P, is calculated based on the standard deviations of distances and angles. The following formulas are used to solve for 
, the weighted distance value, and 
, the weighted angular value:
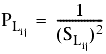
where:
is the standard deviation of the length ij, and
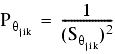
where:
is the standard deviation of angle jik
The final step is to calculate the A matrix, which relates the changes in coordinates to each observation determined through the use of distance observation equations and angle or whole circle bearing/quadrant bearing observation equations. These equations are initially nonlinear, so the Taylor series approximation is used to render them as linear equations.