Results
Examine the results of the temperature dependent problem.
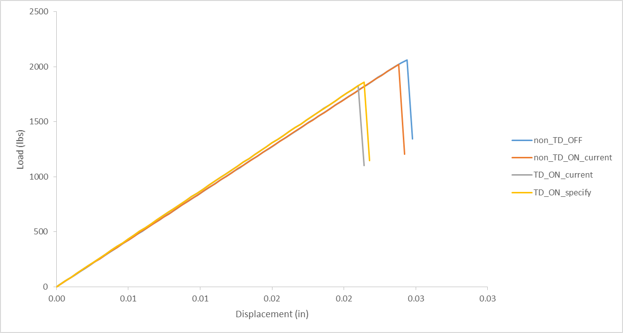
The load-displacement curves for the models are shown in the plot below.
- non_TD_OFF: non-temperature dependent material excluding constituent level thermal residual stresses
- non_TD_ON_current: non-temperature dependent material including constituent level thermal residual stresses calculated using material properties at the current temperature environment
- TD_ON_current: temperature dependent material including constituent level thermal residual stresses calculated using material properties at the current temperature environment
- TD_ON_specify: temperature dependent material including constituent level thermal residual stresses calculated using material properties at a specified temperature environment
All models have very similar stiffness values with the two temperature dependent models (TD_ON_specify & TD_ON_current) having a slightly higher stiffness. These two models have a slightly higher stiffness because the measured E11 and E22 values increase from 72°F to 260°F and these models use properties at 140°F, compared to the other models which use properties at 72°F.
The TD_ON_current model fails at 1827 lbs, the TD_ON_specify model fails at 1861 lbs, the non-temperature dependent model that includes thermal stresses (non_TD_ON_current) fails at 2020 lbs, and the non-temperature dependent model that excludes thermal stresses (non_TD_OFF) fails at 2059 lbs. The TD_ON_current model fails earlier than the two non-temperature dependent models because the interpolated compressive strengths -S11 and -S22 at 140°F are lower compared to the compressive strengths at 72°F.
The TD_ON_specify model, which uses material properties at 72°F for the calculation of thermal residual stresses, fails at a higher level than the TD_ON_current model, which uses material properties at 140°F for the calculation of thermal residual stresses. The calculation for the temperature change in these two cases is different. For the TD_ON_specify model, the cure ratio Rcc is applied to the change in temperature from 72°F to 140°F. Thus, the TD_ON_specify model has lower thermal residual stresses, which correlates to the higher ultimate failure. Refer to the Thermal Residual Stresses topic in the Theory Manual for details on the calculation of the temperature change.