In this Help section, all calculation relations used during the full checking calculation of hydrodynamic radial plain bearings are presented. Calculated relations are listed in the order they are used in the bearing calculation.
The calculation is done in XY and XZ planes and their quadratic sum is made.
Angle speed of bearing journal:
Circumferential speed of bearing journal:
Active bearing width
For bearings lubricated through the lubrication hole or axial lubrication groove
L f = L [mm]
For bearings lubricated through the radial (circumferential) lubrication groove
L f = L - s [mm]
Relative bearing width
For bearings lubricated through the lubrication hole or axial lubrication groove
For bearings lubricated through the radial (circumferential) lubrication groove
Bearing pressure
Values in the range from 1 MPa to 5 MPa are considered for small specific pressures, maximum values are around 30 MPa and 70 MPa during short-time impact loading, but only with the correct selection of journal and bearing material.
The minimum thickness of lubricant layer to ensure the total separation of sliding bearing faces:
for bearings with consideration of running-in
h min = 3.4 (R aH + R aL ) + o [μm]
for bearings without running-in
h min = 4.5 (R aH + R aL ) + o [μm]
If a condition of hydrodynamic lubrication is to be true, the minimum thickness of the lubricant layer must be less than the specified diametral clearance.
Mean hydrodynamically effective relative diametral clearance:
for bearings with consideration of running-in
for bearings without running-in
The calculation of diametral clearance changes due to the pressing of the bearing bushing Δd p and the diametral clearance changes due to radial temperature gradient Δd T are made in the advanced data dialog box.
Relative diametral clearance is an important design parameter, which affects the bearing properties. Its range is 0.0005 ~ 0.004. Small values of relative diametral clearance are suitable for bearings with high specific pressure, working at small sliding speeds and vice versa.
With increasing value of relative diametral clearance the bearing load capacity falls and a risk of journal vibrations and cavitation of the bearing lining increase.
Sommerfeld number
The dimensionless Sommerfeld number is the basic value for considering bearing load capacity. The recommended value is in a range from 1 to 15. Due to the small specific pressure during high sliding speed, a danger of irregular bearing running is possible for a Sommerfeld number lower than 1. For values greater than 15, there is the danger of contact on sliding faces.
Relative journal eccentricity:
The value of relative eccentricity is obtained from the diagram by its dependence on the Sommerfeld number and the relative bearing width.

The recommended value for the relative journal eccentricity ranges from 0.7 to 0.96. For lower values an irregular journal usage. When exceeding the upper limit, maximum friction between peaks of surface roughness can happen.
Minimum thickness of hydrodynamically effective lubrication layer while the bearing is running:
h o = 0.5 φ d (1 - ε 10 3 [μm]
If the condition for hydrodynamic lubrication is to be satisfied, the calculated thickness of the lubrication layer must be greater than the minimum thickness of the lubrication layer.
Bearing thermal balance is made for specified bearing dimensions and selected lubricant.
Check of bearing journal for bending:
Maximum pressure in the lubrication slot:

where: P * cm is a dimensionless specific pressure number in the mean bearing plane, which is taken from a diagram according to specified relative journal eccentricity and relative bearing width
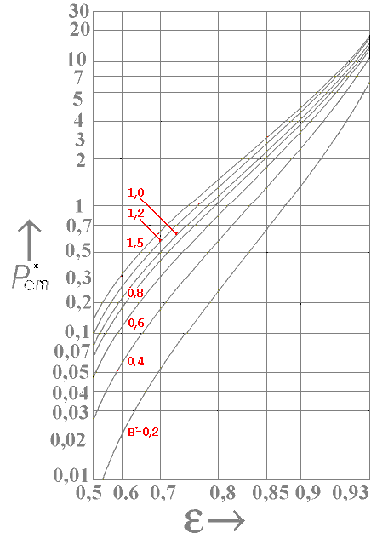
Value of maximum pressure during running and size of specific bearing pressure during start-up and run-out are also obligatory data for the design of bearing bushing material.
Transient speed frequency at the limit of maximum friction:

If bearing friction and wear are extensive because of operating conditions during bearing start-up and run-out, the transient speed frequency must expressively lay under the operating frequency to shorten the period of insufficient lubrication.
Maximum bearing load in the limit of maximum friction:
Maximum speed frequency at the limit of turbulence rise:

where:
π t - π 20 - 0.65 (T - 20) [kg m -3 ]
Maximum speed frequency at the limit of journal whirling:
